Breve descrição
A flask with water serves as an example of the sea that is irradiated. In the experiment, the Sun is replaced by a strong lamp that injects heat into the water. The expansion of water is monitored via a water column inside a glass tube or capillary tube. The qualitative observation is discussed and augmented by calculating the expansion based on simplified assumptions.
This resource is part of the educational kit "The Climate Box". You can read more about the kit in the presentation attached. Find all related resources selecting the category "Our fragile planet" and "secondary level".
Objetivos
With this activity, the pupils will learn that water expands when heated. When translated to the situation on Earth, they will realise that also the oceans expand when they absorb heat, which contributes the global rise of sea levels.
Objetivos de aprendizagem
After this activity the students will be able to
- explain that water expands when heated.
- explain that the oceans are rising because of the rising temperatures of the atmosphere and the water.
Avaliação
- During the activity the teacher should guide the students to the connection between heating and the rising water level.
- The teacher should ask the students to carefully observe and describe the result of the experiment.
- During the activity, the students calculate the effect of volume increase of water when heated.
Material
Indicated is a set of items needed for one experimental set-up. Multiply by number of experiments to be carried out in parallel.
- Round flask (250 ml, narrow neck)
- Rubber stopper (Ø 29 to 35 mm) with bore hole (Ø 7 mm)
- Some glycerine as lubricant
- Glass tube with small diameter (8 mm, length: 250 mm)
- Bright lamp or spotlight
- Water
- Thermometer
- Water soluble felt pen
Informações de referência
Sea surface altimetry
One of the most challenging consequences of the ongoing climate change on Earth is the rise of the sea levels that poses a threat to many coastal areas. Monitoring sea levels used to be a quite difficult task, as until a few decades ago, this could only be done via direct measurements and probes on open seas. With the advent of earth observing satellites and radar altimetry missions, it became possible to monitor sea levels very efficiently and effectively. While in situ measurements only provide a very local perspective of a given probe and the resolution of the global network is rather low, satellite measurements allow observing the entire earth at a high redundancy and frequency with at the same time high spatial resolution.
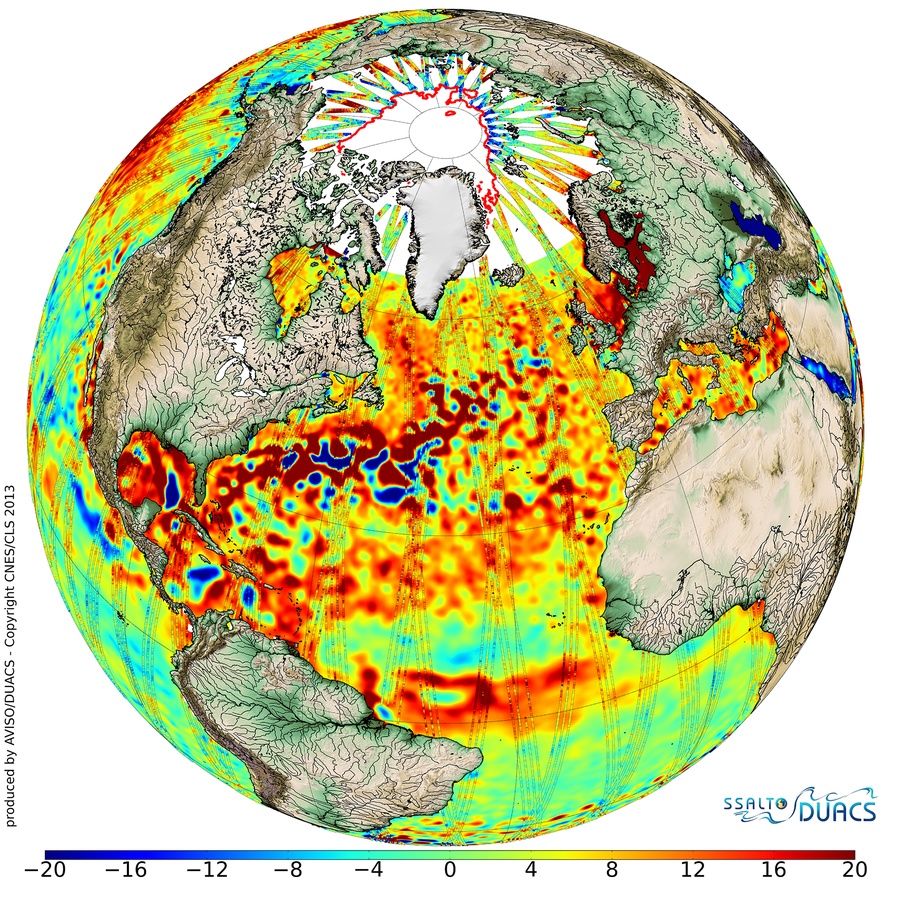
Figure 1: Average sea-surface topography for 2013 as mapped by CryoSat combined with data from other missions. Red represents higher sea levels (up to 20 mm above average) while blue represents lower areas (down to –20 mm). The perturbations seen in the Northern Atlantic are caused by the warm Gulf Stream current (Credit: ESA/CNES/CLS, http://www.esa.int/spaceinimages/Images/2014/06/2013_sea-surface_topography).
Known satellites with altimetry capabilities are e.g. CryoSat and Jason 2/3. The most recent addition is Sentinel 3, a flagship of the European Copernicus Earth observing programme that offers an unprecedented spatial resolution.

Figure 2: Sea level in 2014 compared to the global average at the mid-point of the 1993-2013 time series (Credit: NOAA Climate.gov map, adapted from Figure 3.25a in State of the Climate in 2014 report, https://www.climate.gov/news-features/understanding-climate/2014-state-climate-sea-level).
Oceans are on the rise
When combining such data obtained at different acquisition times, a general trend of the sea level evolution can be derived. When analysing all the data at hand, it is obvious that sea levels have been rising during the last one and a half centuries (Figure 3).
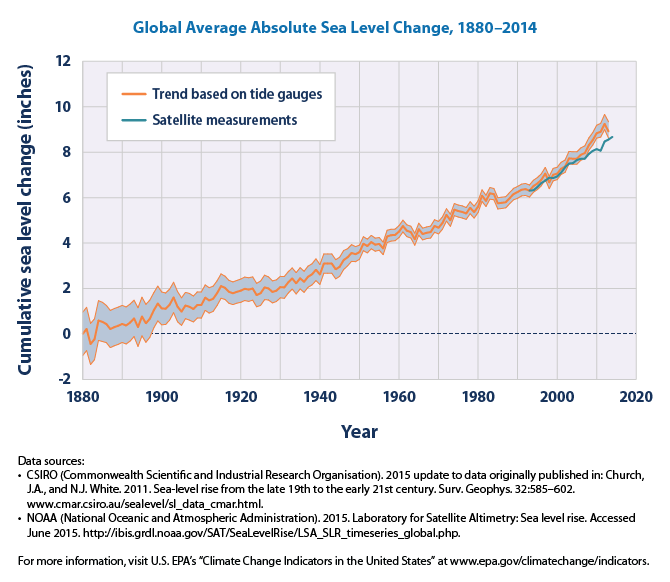
Figure 3: This graph shows cumulative changes in sea level for the world’s oceans since 1880, based on a combination of long-term tide gauge measurements and recent satellite measurements. This figure shows average absolute sea level change in inches (1 inch = 25.4 mm), which refers to the height of the ocean surface, regardless of whether nearby land is rising or falling. Satellite data are based solely on measured sea level, while the long-term tide gauge data include a small correction factor because the size and shape of the oceans are changing slowly over time. (On average, the ocean floor has been gradually sinking since the last Ice Age peak, 20,000 years ago.) The shaded band shows the likely range of values, based on the number of measurements collected and the precision of the methods used (Credit: United States Environmental Prtotection Agency, https://www3.epa.gov/climatechange/science/indicators/oceans/sea-level.html).
In summary, current scientific results show that from 1993 until 2014 the ocean levels have been rising with a rate of up to (2.9 ± 0.4) mm per year. That is 6 cm within 20 years. And the rate seems to be increasing.
Thermal expansion of liquids
When heated, liquids expand isotropically. This is the principle of the common thermometer. With a constant pressure and a constant amount of substance the relative volume increase ΔV/V is sufficiently described by the following linear differential equation.
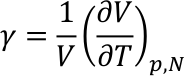
The quantity γ is the so called cubic expansion coefficient with the unit K-1. If γ does not change with temperature, we can write γ(T)=γ(T0), and the linear differential equation can easily be solved. This is actually incorrect, but a good approximation for small temperature changes.
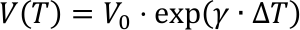
For small temperature variations, a Taylor expansion results in:
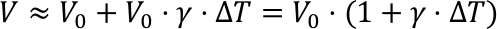
With these properties the temperature dependence of density can be explained. As the mass does not depend on the temperature, the following equation is valid:

With m being the constant total mass. Since the volume V changes with temperature, the density ϱ does as well. In reality, the cubic expansion coefficient is a function of temperature. Therefore, the actual value must be selected, even if the variations around the given temperature are small. This means e.g.:
Table 1: Selection of values for the volume expansion coefficient of water for a set of temperatures (from Grigull et al. (1990), https://en.wikipedia.org/wiki/Water_(data_page)).

¹Boiling point of water at standard conditions, i.e. ambient pressure p = 1000 hPa according to “Formulation of the Thermodynamic Properties of Ordinary Water Substance for Scientific and General Use", International Association for the Properties of Water and Steam (1984).
For ϑ = 0°C, γ is negative. This is a consequence of the density anomaly of water. Pure water in its liquid form attains its highest density at sea level conditions at a temperature of 4°C. It expands when lowering its temperature to the freezing point.
The connection to sea level rise
This behaviour of water is one possible contribution to the observed sea level rise, in particular when considering the global warming and the role of the oceans as a heat sink (see corresponding activities of the EU Space Awareness suite). Current research amounts the contribution to the global sea level rise to a rate of about 0.5 mm per year between 1955 and 2010.

Figure 4: Cumulative temporal evolution of the sea level change caused by heat expansion (thermosteric sea level change) between 1955 and 2015 (http://www.nodc.noaa.gov/OC5/3M_HEAT_CONTENT/).
Descrição da atividade completa
Activity 1: See the water rise
The major part of the sunbeams is absorbed by the surface of the Earth, and in particular by the oceans in form of heat. Target of this experiment is to examine the effects of this absorption.
Let the students discuss the following questions.
Q: What are the characteristics of a body, a liquid or a gas when warming-up? Have you seen a balloon filled with air and then heated? A: Bodies, liquids and gases can expand when heated.
Q: When dealing with water, is this effect rather strong or small? Can you observe an obvious rise of the water level when heating water in a pot (before boiling)? A: The effect must be small. The change cannot be observed easily by naked eye.
Q: What does this effect mean for the oceans on Earth? A: This means that the water in the oceans of the Earth expands when heated.
Experimental set-up
- The flask is completely filled with water.
- Measure the temperature of the water with a thermometer.
- Close the tube including the glass tube or capillary tube with the stopper avoiding air bubbles. The water level in the glass tube should be visible.
- Mark the water level on the glass tube with the overhead marker.
- Position the lamp at a distance of approx. 5 cm in front of the flask.
- Shield the glass tube to avoid heating the water inside.

Figure 5: Experimental set-up.
Procedure
Switch on the lamp and observe the liquid in the glass tube for about 10 minutes.
Q: By how much does the water rise in the glass tube? A: The water level rises by several millimetres corresponding to a volume increase of only a few cubic millimetres. Compared to the total volume the rise is rather small.
Let the students write down their observations and discuss the results. What conclusions can be drawn for the Earth, especially for the oceans?
Result: Due to the illumination with the lamp the temperature of the water increases and the water level in the glass tube rises, i.e. it expands. Liquids generally expand with increasing temperature.
Transferring this scenario on the situation on Earth, this means heating the oceans leads to a rise of the sea levels.
Calculate it! (optional for term 7 and higher)
The chart below shows the density of liquid fresh water vs. its temperature. Note that the values for salty sea water differ. They depend on its salinity. However, the density of sea water is higher and the expansion effect is stronger. Individual values can be derived from the online calculator: http://www.csgnetwork.com/h2odenscalc.html
Table 2: Densities of fresh water at standard pressure for given temperatures (according to NIST, https://is.gd/joDUoD).
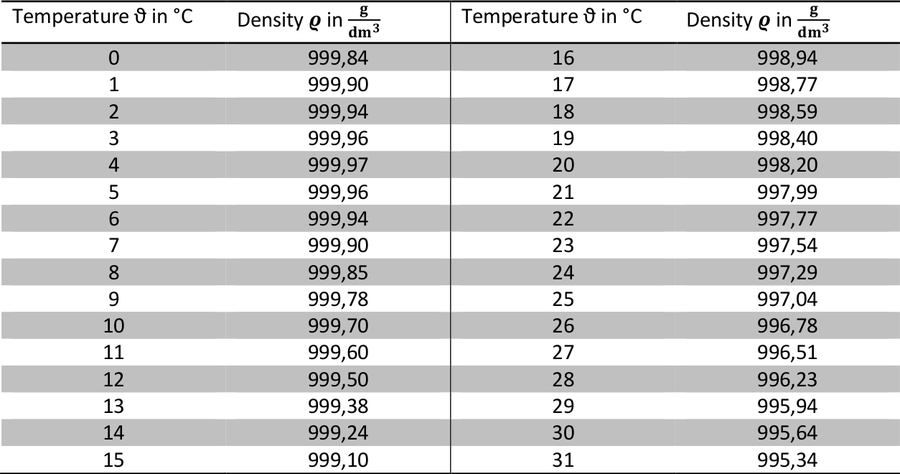
- 1 litre (= 1 dm³) of water is heated from 10°C to 20°C.
- What is the volume of the water after warming? Note that the total mass of the water does not change.
- What is the increase of the volume in percent?
The average sea depth on Earth is approx. 3 800 m.
- What is the volume of a water column in dm3 of a cuboid with a base area of 1 m2 and a height of 3 800m?
- Provided the temperature of the ocean would have an average of 5°C. What would be the volume of the water with a temperature increase of 1°C?
By how much does the sea level rise?

Figure 6: Sketch of a water column with a square base area of 1 m x 1 m and an altitude of 3 800 m.
- What does that mean for the rise of the sea levels by storing heat due to global warming?
Note that only the upper few hundred metres of the oceans are actually heated by insolation. Therefore, the real consequences are smaller.
Solutions
- 1 litre (= 1 dm³) of water is heated from 10°C to 20°C.

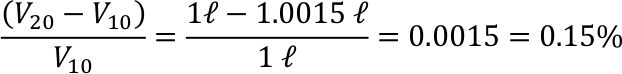
The volume is increased by 0.15%.
- The average sea depth on Earth is approx. 3800 m.

- The average sea depth on Earth is approx. 3800 m.
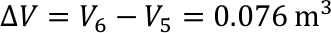


Currículo
Space Awareness curricula topics (EU and South Africa)
Our fragile planet, oceans, climate change
This activity is part of Space Awareness category “Our fragile planet” and related to the curricula topics:
- Composition and structure
- Oceans
- Climate change
- Surface
Informações adicionais
This activity is part of a larger educational package called “Our Fragile Planet – The Climate Box”.
Conclusão
The activity involves a simple experiment that demonstrates how water expands when heated. It can be understood as a model for the oceans on Earth that absorb large amounts of heat. The so called thermosteric, i.e. caused by warming, sea level rise is a minor, yet measurable contribution to the global rise of ocean levels. The students investigate the processes both qualitatively and quantitatively. The latter is suggested for higher terms and uses simple calculations of given examples.

This resource was developed by Marco Türk, Heidelberg University and Markus Nielbock, Haus der Astronomie, Heidelberg, Germany. This resource is under peer-review, proof reading, and will be updated and improved in the coming year.

